
1 Introduction
In the first part of this article, we sketched a general framework to calculate the bank’s value. In this second part of the article, we will show how to apply the framework to the evaluation of a contract that is inserted in the existing bank’s balance sheet and how to properly compute the xVAs quantities. Finally, we will see how to conciliate the apparently theoretical unsound market practices to evaluate derivative contracts, and the nowadays standard results of the modern financial theory, namely the Modigliani-Miller (MM) theorem (see Modigliani and Miller, [3]).
An extended version of this work, with the details of the analytical results, is available at www.iasonltd.com in the research section.
2 A Non-Trivial Set-Up to Evaluate Contracts
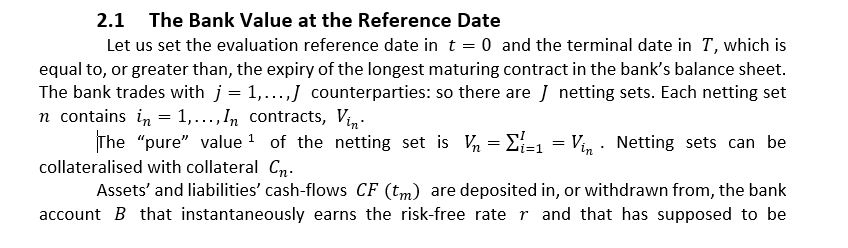
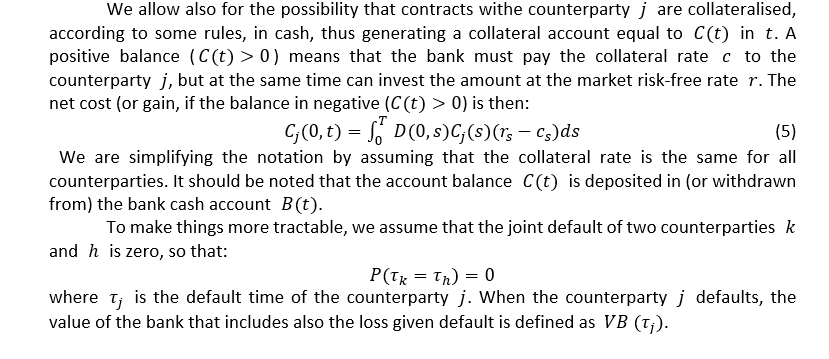
We can specify the general framework sketched in the first part to evaluate the incremental contribution of a contingent claim in the balance sheet of the bank. First, we outline how to calculate the value of the bank; then, we will assess how the insertion of a new contract in the bank’s balance sheet impacts the value.




3 Incremental Valuation of a New Contract


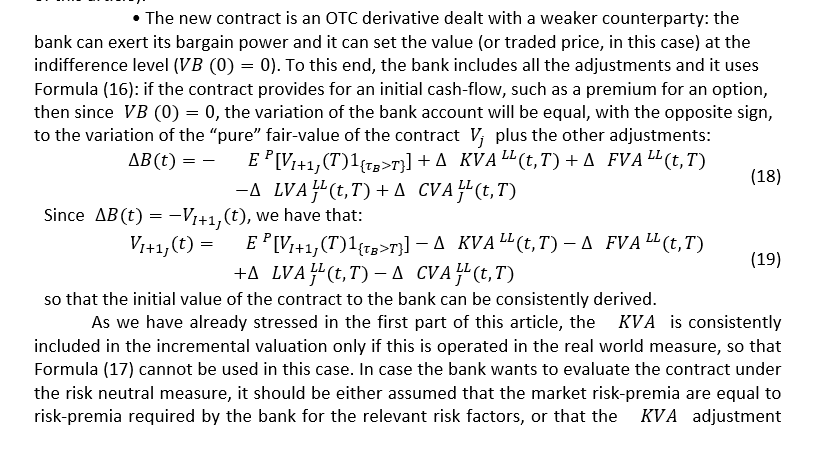

4 Reconciliation with the Modigliani&Miller Theorem
Elsewhere,[1] we had to opportunity to stress that the incremental valuation framework that we introduced above is not in contrast with the main tenet of the Modigliani&Miller (MM) theorem, expounded by the two authors in their article of 1958 (see [3]).[2] On the contrary, when evaluating an investment that is included within the balance sheet of a company (bank) that has already started its operations, then the only way to keep the total value of the assets of the company equal to the total value of the liabilities, is to apply the principles of the incremental valuation stated in Castagna [2] to the non trivial framework sketched above.
In the recent work by Andersen et al. [1], the Modigliani&Miller theorem is proved to be correct when calculating the incremental value of a contract with respect to the total firm value, which is equal to the total value of the assets. In this case, the authors prove that the correct incremental value is given by the “pure” value, deducted of the CVA and incremented by the DVA, and it is independent from the way it is financed.
In our framework, we calculate the value of a contract only with respect to the value of the bank to the shareholders, because we think this is the only meaningful way the indifference to inclusion of the contract in the balance sheet to all stakeholders. When considering the total value of the firm, the evaluator allows for wealth transfers from shareholders to claimants of higher order, such as bondholders (see Andersen et al. [1], pag. 159). On the contrary, when considering the incremental value with respect to the shareholders’ bank value, no wealth transfer is allowed and the contract value is such that all claimants are indifferent to it. Sure, such a value entails additional costs that have to be paid by the counterparty, but here we enter in the market action, where the price of the contract is determined. The price can be set at a level that matches the internal incremental valuation of the bank, thus generating a nil net contribution to the bank value; or it can be different, with a net positive or negative contribution. In any case, the price setting is the result of the bargaining process where the strengths of the bank and of the counterparty clashes and, possibly, they eventually agree to close the deal.
We think that the approach that we have detailed above and that relies on the simpler, but in any case complete, setting in Castagna [2], is in line with Proposition III of Modigliani and Miller [3], where the the optimal investment rule is derived: basically, when the firm (i.e.: the manager) is acting in the shareholders’ best interest, it will undertake an investment only if its rate of return is at least equal, or above the rate of return required by the market for a class of risk corresponding to the riskiness of the firm. In our approach, we are internally setting the rate of return of a new contract by adding all the adjustments that make its rate of return equal to the appropriate rate of return. The latter is determined by the current composition of the assets and their related risks, and by the debt and equity capital financing it, whose costs mirror the leverage and the risk premium above the risk-free rate requested by the debt-holders and shareholders.
In our opinion, in Modigliani and Miller [3] it is Proposition III that has a normative value and that should be considered when designing a framework to evaluate new contracts. Proposition I and II, in the same article, have a positive value describing the equilibrium that can be retrieved ex post, equating the return of the assets to the average cost of capital, whichever mix the firm chooses to finance them. But both propositions are not including investments that produce a loss of wealth of one of the stakeholders in favour of another stakeholder: these investments are clearly excluded by Proposition III. Following the latter, we were able to derive the rules that determine the hurdle rate at which the actual contribution of contract to the (shareholders’ bank value) is nil. It is clear that accepting only the investments that comply with Proposition III, also Proposition I and II will be proved to be true, provided we are working in a frictionless, perfect financial market.
5 Conclusion
In this work we have extended the approach of Castagna [2] to a non-trivial setting to calculate the incremental value of a contract that is included in the bank’s balance sheet. A similar approach has been recently developed by see Andersen et al. [1]. To our knowledge, our framework is richer than those appeared since now in literature, in that we include a firm structural framework within a classical general equilibrium framework.
The framework considers different financing policies and consistently derives all the adjustment to the “pure” value of a contract, including the CVA , the FVA and, implicitly, the LLVA . We are also able to derive, in a natural fashion, an adjustment that relates to the KVA. In our structural, general-equilibrium enhanced framework setting, we do not only flesh out the origin of the KVA, but we can also identify the cases in which its inclusion is admissible in the evaluation, which is the correct premium to consider and, moreover, we can spot potential double counting of the adjustment.
References
[1] L Andersen, D. Duffie, and Y.. Song. Funding value adjustmentss. Journal of Finance, LXXIV(1):145–191, 2019.
[2] A. Castagna. Towards a theory of internal valuation and transfer pricing of products in a bank: Funding, credit risk and economic capital. Iason research paper. Available at http://www.iasonltd.com, 2013.
[3] F. Modigliani and M.H. Miller. The cost of capital, corporation finance and the theory of investment. The American Economic Review, 48(3):261–297, 1958.
[1] See Castagna [2].
[2] We would like to recall here the MM theorem proves that the value of a project is independent from the way it is financed, or from the capital structure of the company undertaking it.



